【每日力扣】437. 路径总和 III 与105. 从前序与中序遍历序列构造二叉树

🔥 个人主页: 黑洞晓威
😀你不必等到非常厉害，才敢开始，你需要开始，才会变的非常厉害

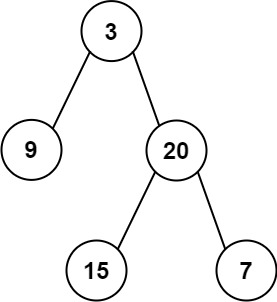
437. 路径总和 III

给定一个二叉树的根节点 root ，和一个整数 targetSum ，求该二叉树里节点值之和等于 targetSum 的 路径 的数目。

路径 不需要从根节点开始，也不需要在叶子节点结束，但是路径方向必须是向下的（只能从父节点到子节点）。

解题思路

1. 由于路径不必从根节点开始，也不必在叶子节点结束，所以对于每个节点，都要尝试以该节点作为路径的起点，向下搜索满足条件的路径数量。
2. 递归遍历节点，并分别计算包含当前节点以及不包含当前节点的路径数。
3. 对于每个节点，递归计算包含当前节点的路径数量，然后递归计算不包含当前节点的路径数量。
4. 统计符合条件的路径数量。

代码实现

class Solution {
    public int pathSum(TreeNode root, int targetSum) {
        if (root == null) {
            return 0;
        }
        
        // 以当前节点为路径起点的路径数 + 以左子树为路径起点的路径数 + 以右子树为路径起点的路径数
        int pathsFromRoot = countPaths(root, targetSum) + pathSum(root.left, targetSum) + pathSum(root.right, targetSum);
        
        return pathsFromRoot;
    }
    
    private int countPaths(TreeNode node, int targetSum) {
        if (node == null) {
            return 0;
        }
        
        int count = 0;
        if (node.val == targetSum) {
            count++;
        }
        
        count += countPaths(node.left, targetSum - node.val);
        count += countPaths(node.right, targetSum - node.val);
        
        return count;
    }
}

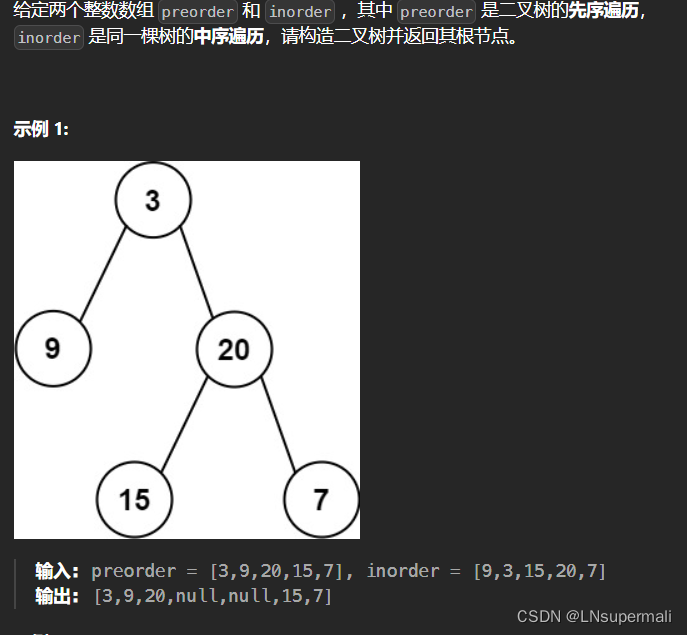
105. 从前序与中序遍历序列构造二叉树

给定两个整数数组 preorder 和 inorder ，其中 preorder 是二叉树的先序遍历， inorder 是同一棵树的中序遍历，请构造二叉树并返回其根节点。

解题思路

1. 先序遍历的第一个元素为当前树的根节点。
2. 在中序遍历中找到根节点的位置，左边为左子树的中序遍历，右边为右子树的中序遍历。
3. 递归构建左子树和右子树。

代码实现

class Solution {
    public TreeNode buildTree(int[] preorder, int[] inorder) {
        return buildTreeHelper(preorder, inorder, 0, 0, inorder.length - 1);
    }
    
    private TreeNode buildTreeHelper(int[] preorder, int[] inorder, int preStart, int inStart, int inEnd) {
        if (preStart > preorder.length - 1 || inStart > inEnd) {
            return null;
        }
        
        TreeNode root = new TreeNode(preorder[preStart]);
        int inIndex = 0;
        for (int i = inStart; i <= inEnd; i++) {
            if (inorder[i] == root.val) {
                inIndex = i;
            }
        }
        
        root.left = buildTreeHelper(preorder, inorder, preStart + 1, inStart, inIndex - 1);
        root.right = buildTreeHelper(preorder, inorder, preStart + inIndex - inStart + 1, inIndex + 1, inEnd);
        
        return root;
    }
}